Mathematics is everywhere – a visit to the world of Alessio Figalli
Alessio Figalli is a recipient of the Fields Medal for his research into optimal transport theory. Who is this individual? What characterises his research? In what way is mathematics a creative thought process? How are clouds, soap bubbles and crystals related to transport costs? The portrait of a mathematician who has made problem-solving his profession.
Mathematics is everywhere. You only have to see it, says Alessio Figalli and looks at the sky. Clouds are moving across the city of Zurich. Some are large, some small. None keeps its shape for long. It's these shape changes that interest Alessio Figalli. They offer him the perspective to understand the underlying mathematics; the mathematics for which he received the Fields Medal, the ultimate accolade for a mathematician under the age of 40.
Mathematics is everywhere. You need creativity to be able to see that, says Figalli, making his way to his office in the main ETH building. Figalli likes walking: it's a good way of finding fresh ways of looking at a mathematical question, especially when the problem is one that's been preoccupying mathematicians for years – or even longer. "To be the first to solve an open question, you have to develop your own ideas. You then try to combine the results from different areas and come up with new techniques for tackling the problem. That's a creative thinking process," says Figalli.
Secret of the clouds
When it comes to clouds, he hit the jackpot: together with other mathematicians, Figalli was able to demonstrate that certain changes in the shape of clouds can be calculated mathematically as an optimal transport problem. In other words, the same – or related – equations can be applied to clouds as are used to calculate the most favourable transport route for resources or people.
The solution idea: when one cloud changes shape abruptly, countless cloud particles move from one position to another. This activity can be visualised as particles being transported from one place to another, and is something that applies, in particular, to the cloud formations of a large weather front. These often change so abruptly that describing them mathematically is a real challenge.
The relationship between transport optimisation and meteorology had already been known since the 1990s, but Figalli only began to tackle this "semi-geostrophic problem" in 2005. However, important results were missing in detail. He set out to investigate the highly complex differential equations and their properties and dependencies.
Differential equations describe movements and changes. They are one of the key tools of the natural and engineering sciences and a prerequisite for many technical achievements of modern society. A well-known differential equation is the Monge-Ampère equation which, in essence, describes the most efficient way of transporting objects or resources.
Gaspard Monge (1746-1818) was a French mathematician and government minister under Napoleon. He wanted to know how building materials could be transported to the construction sites in order to build fortifications as cost-effectively as possible. Or to put it another way: assuming the transport costs are to be kept as low as possible, which sites should the various materials be taken to?
The cost of change
Translating to the clouds: if you know that the particles being moved from the initial distribution to the final distribution minimise their "transport cost", you can calculate the optimal path. This yields information on how the clouds change their shape.
Figalli and his colleagues investigated the Monge-Ampère equation for seven years before making a breakthrough in 2012: they were able to prove new fundamental results on the properties of the solutions. They also showed that the equation could be used to describe changes in cloud formations.
"In applying the theory of optimal transport to physical and chemical phenomena, the equation plays an important role," Figalli explains. "In many cases the concept of minimal 'cost' refers to energy, because nature minimises energy."
Aside from clouds, Figalli has also looked at changes in the shape of soap bubbles and crystals. Both bubbles and crystals strive for a shape that keeps their surface energy as low as possible. The types of energy are different at a physical level, but mathematically the equations are very similar. The theory of optimal transport can be applied to both phenomena to describe how their shapes change when energy is supplied. After that, it's possible to study how the particles are transported from the configuration with minimal energy to the one with increased energy.
Original thinker and problem-solver
Some mathematicians develop new techniques destined to open doors to entirely new fields of research: this was the case when Isaac Newton (1642-1726) and Gottfried Wilhelm Leibniz (1646-1716) introduced differential calculus. Alessio Figalli is not one of them. Rather than design new theories and models, he concentrates on developing solutions to existing problems – preferably those that mathematicians have been studying for a long time.
In this competition, anyone aspiring to be the first to solve a problem and support it with bullet-proof evidence requires a great deal of creativity and originality in addition to profound knowledge. This includes accepting dashed expectations: "It's a long way from the idea to the rigorous proof. I've failed many times, but I've always learned something from it," says Figalli. He was fortunate enough to have amongst his teachers some of the most original and innovative mathematicians: Luigi Ambrosio in Pisa, Italy, Cédric Villani in Lyon, France (Fields Medal holder in 2010), and Luis Angel Caffarelli in Austin, Texas/USA.
Figalli was a professor in Austin for seven years before he came to ETH; people there called him an "ace problem-solver". Figalli is something of a sportsman, preferring to play basketball and football in his leisure hours. He's married and describes himself as a reliable and efficient researcher. Mathematics, he says, emerges from dialogue.
He values the quality of the students and the vibrant, dynamic environment at ETH Zurich, and likes the fact that there is another strong institute of mathematics at the University of Zurich.
Meteoric career after a late start
His career trajectory has been stellar: after studying at the Scuola Normale Superiore in Pisa, a talent factory where student selection is based exclusively on merit, he completed his doctorate in just one year. When he graduated he was immediately an assistant professor in Nice. Aged just 25, he was made an associate professor at the University of Texas in Austin, USA where he became a full professor at age 27. He was 32 when he relocated to ETH in 2016.
Figalli did not have an early vocation for mathematics: his passion for the discipline was sparked only when at high school a teacher persuaded him to take part in the Mathematics Olympiad. His father was an engineering professor, so he grew up thinking that mathematics was a tool for engineers. It did not occur to him that it could be a discipline in its own right. Today, he's keen to "show young people that mathematics is a creative profession."
Mathematics is everywhere.

References
Figalli A, Guionnet A: Universality in several-matrix models via approximate transport maps. Acta Math. 217 (2016), no. 1, 81-176.
De Philippis G, Figalli A: Partial regularity for optimal transport maps. Publ. Math. Inst. Hautes Études Sci. 121 (2015), 81-112.
De Philippis G, Figalli A: W^{2,1} regularity for solutions of the Monge-Ampère equation. Invent. Math. 192 (2013), no. 1, 55-69.
Ambrosio L, Colombo M, De Philippis G, Figalli A: Existence of Eulerian solutions to the semigeostrophic equations in physical space: the 2-dimensional periodic case. Comm. Partial Differential Equations 37 (2012), no. 12, 2209–2227.
Figalli A, Maggi F, Pratelli A: A mass transportation approach to quantitative isoperimetric inequalities. Invent. Math. 182 (2010), no. 1, 167-211.
Background information for the media

Further background information, photo material, a short film and video statements by 2018 Fields Medal Winner Professor Alessio Figalli (EN, FR, IT) and Professor Lino Guzzella, President of ETH Zurich (DE, EN, IT), can be found at www.ethz.ch/fields-medal-for-figalli .


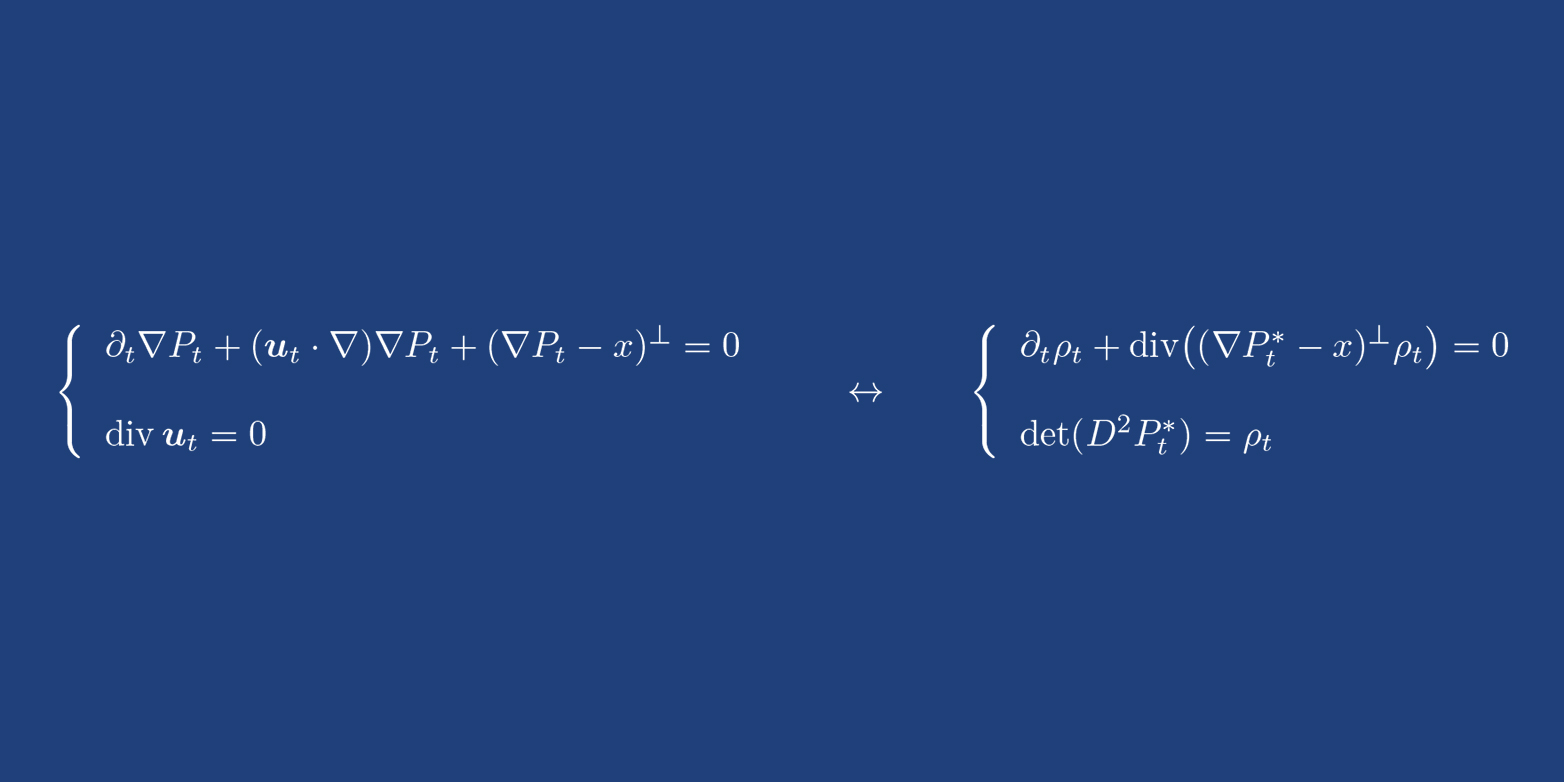

Comments
No comments yet